 |
 |
Maybe some of it is written in error; . . . or much of it is written in error? My challenge is for those who read it and dispute parts of it to enlighten me as to what parts of this writing I need to change to make it all correct, if they have a better understanding of horseshoe pitching, physics and mathematics! I was not a physics major or math major in college. I can only apply simple logic, common sense, and some of my own instincts for expressing my own thoughts, along with some humor thrown in to keep it a little on the light side. But by golly, with this writing, I’ve started the dialogue and anyone willing to engage in it with me, will be a part of the dialogue and will help me create a more perfect writing that may just get a lot more people interested in our sport, who found the idea of figuring something out in the mind first and then applying that knowledge in practice exciting; before feeling total frustration in finding that they have no clue when only engaging in trial and error on the horseshoe courts. How many times does the average pitcher go from hitting ringers to missing and then in error change the wrong thing in trying to tune their pitching.
I have numbered the paragraphs for easy reference so other horseshoe pitchers or physics students or mathematics students can refer to portions when commenting. Let the writing begin and may only those who wish to find enlightenment actually find it! PS: This last summer, I pitched in the 25% range, but had no clue what I was doing wrong. Like other pitchers, I got into rhythms where I would hit up to 6 ringers in a row or throw 14 ringers out of 24 shoes at 58%, but still didn’t know what I was doing right and soon started doing too many things wrong. This writing represents winter thought to prepare for the next summer season.
CHALLENGE ACCEPTED!
In August 2008, I received an address link for another web site blog
dedicated to finding the perfect horseshoe swing delivery. Bob Rasmussen
writes in detail about many of the things discussed on these pages, plus
he takes it a step further by explaining the equipment he has developed
to assist in training the muscles to duplicate the same motion over and
over. His blog web site is located at:
The contents of this study grew too large to be contained on only one page, so on 10/05/05 it was broken up into two separate pages: "Part 1 Essential Basics" and "Part 2 Deeper Speculation". At the bottom of this page is a section labeled "Sections of Part 1 page".
Highlighted in red print are sections that have been added or revised since the original writing was put up on the Internet.A DROPPED SHOE AND THE DISCOVERY
OF GRAVITY
2 If a horseshoe is held in
front of the pitcher and dropped, it will likely fall straight down and
hit the pitcher’s foot. Gravity keeps a horseshoe from being suspended
in air after it is released.
FORWARD MOTION OF THE HORSESHOE
3 It takes a forward motion
on the shoe, while holding it in the hand, to get it away from the pitcher
before it lands; thus making it much safer for the foot.
STRAIGHT TOWARDS THE STAKE FOR RINGERS
4 To have a better chance
of going around the stake at the end of the court 37 feet away, (which
by the way is the object of horseshoe pitching), it would be preferable
for the forward motion to be straight towards the stake at the opposite
end of the court. That is the only direction that gives the shoe
a chance of being a ringer (at least on the court the pitcher is pitching
on). Optimistic note here: A two and a half pounds hunk of
metal goes exactly where you throw it without wandering off on its own.
(note: the maximum weight of a shoe is two pounds and ten ounces.)
IMPORTANCE OF THE BACK SWING
5 It is much easier to put
forward motion on the shoe (while holding it) if the pitcher swings the
attached pitching arm and hand straight back behind to a point where it
feels comfortable to let it swing back forward with gravity on the shoe
bringing it back to the side of the pitcher. It will be only natural
at this point for the shoe, with momentum to swing on up, to continue its
forward motion up to about waist level (or a little above or a little below
waist level (3 times world champion Dan Kuchcinski has said between the
knee and the waist)--some pitchers may prefer closer to chest level), at
which point the pitcher will want to release the shoe if it is to have
a chance to go straight for the stake that is 37 feet away (from the foul
line) on the same court the pitcher is pitching on.
GREETING THE STAKE AND STARTING THE
BACK SWING
6 It is much easier to put
back motion on a horseshoe, if the pitcher first holds the shoe up out
in front of the pitcher’s face or at least out in front. While it
is out in front of the pitcher’s face, the pitcher might as well take advantage
of the position of the shoe and do what is called greeting the stake and
that means a point on the shoe or maybe inside the shoe should be aimed
somewhere in the direction of the stake in question (or the stake that
the pitcher would like to see the shoe go on as a ringer). Now that
the shoe is being held in front of the pitcher and out away from the pitcher’s
body (this does take a level of strength with a shoe that weighs around
two and a half pounds), the relaxing of some of the muscles in the arm
a little allows the shoe to naturally start into a downward back swing
motion (again due to gravity), which we know from above will result in
the shoe reaching a point somewhere behind the pitcher where it feels more
comfortable and natural to let the shoe begin to move into a forward motion.
RELEASING THE SHOE INTO FLIGHT
7 Once the shoe reaches that
position out in front of the pitcher around waist level, where it only
seems natural to let go of it before it goes on around and pulls an arm
out of its socket or causes the pitcher to fall backwards; it is a good
idea to release the shoe. Once the shoe is released, it is in flight.
A shoe in flight can be a beautiful thing to behold, but then it is not
always such a beautiful thing to behold (it all depends on the motion or
the lack of motion the pitcher applies to the shoe during the forward swing).
It is either going directly straight at the opposite stake, in which case
it must have been released at about knee level and must be traveling very
fast in order to hit a ringer before gravity lands it somewhere between
the pitcher and the stake; or hopefully the pitcher released it at about
waist level, giving the shoe a thing called “arch”, in which case the upward
motion from the knee on up will cause the shoe to head for a point over
the pitcher’s head and safely out in front of the pitcher and not directly
over the pitcher’s head (in which case something was not done quite properly).
The shoe should reach peak height somewhere short of halfway down the court
or about 20 feet from the stake (according
to one source the shoe peaks in height at about 30 feet down the court
or 10 feet from the stake, but a study of "trajectory" using Google on
the Internet would seem to contradict this assertion). (Another source
said the shoe should be thrown at a height that makes it come in at a 45
degree angle and throwing it 10 feet high causes that to happen.
But if my calculations are correct with a shoe release at about 2-1/2 feet
just above the knee, it would have to reach a peak height of 19 feet going
up at a 45 degree angle in order to come down to a point a few inches above
the ground on the stake. So I'm not sure how they calculated this,
unless they were going with the 10 feet high at 30 feet down the court
theory, which doesn't sound like good physics.)STUDIES
IN TRAJECTORY SEEM TO INDICATE THAT GRAVITY ONLY AFFECTS THE VERTICAL MOTION,
BUT NOT THE HORIZONTAL MOTION OF A PROJECTILE! (Albert Einstein had
some things to say about this with his examples of two people playing catch
with a baseball on top of a moving train and how it appeared relative to
observers on the ground versus observers on top of the train).If
it reaches peak height much farther than halfway (remember, the shoe is
released at about waist level from the ground and will land about 3 inches
from the ground), it will likely either go over the stake or clear the
fence and hit a spectator. It was stated by 3 times world champion
Dan Kuchcinski on his excellent instructional video on horseshoe pitching
titled "Yes, Horseshoes" that a shoe should be thrown at a peak height
of at least a foot over the pitcher's head (I'm 5 feet-7 inches tall, so
I would want to throw for a minimum height of at least 6 feet- 7 inches)
and possibly another foot or two above that (or 7 feet-7 inches or so for
me). He also states that 10 feet is generally regarded as the maximum
height for any pitcher to reach in flight. Above that height the
shoe is said to be harder to control.
PEAK HIGH POINT OF THE SHOE
8 The peak high point is where
the shoe quits going up as it is going forward and begins a descent (again
due to the pull of gravity) while it is still going forward. If the
proper turn rotation motion was put on the shoe, then it will look pretty
during its flight. If the pitcher had no clue where the center of
gravity is on his shoe, didn’t know the shoe had a center of gravity, doesn’t
know what he would do differently if he knew a shoe had a center of gravity
and did not pivot his turn rotation motion around this center of gravity,
then we could either have a shoe that is trying to reach a state of balance
(not a pretty sight) or a shoe that is in total free fall and will never
reach a state of balance during its short flight (May Day, May Day, . .
. flight to ground control).
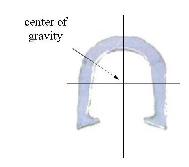
WHERE IS THE CENTER OF GRAVITY OF A HORSESHOE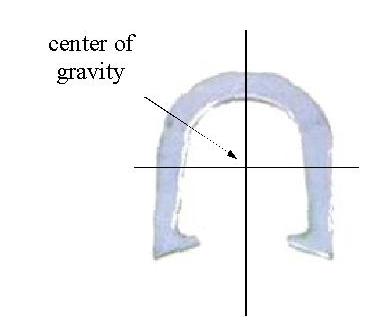
9A A shoe is designed so that when you draw a straight line down
the middle of the shoe from the center of the closed end to the center
of the open end, both halves of the shoe weigh the same. There is
additional weight in the heel calks of the shoe to make up for the lack
of metal in the open end so that there is a place somewhere in the middle
of one blade such that if you draw a line to the same place in the middle
of the other blade, there is the same amount of weight of metal on the
part of the shoe with the closed end as there is of metal making up the
open end of the shoe. (Note: extremely heavy calks, as with
some shoes designed for flip throwers, would move the center of gravity
lower towards the open end of the shoe). Where these two lines cross
is the center of gravity of the shoe. All other lines drawn through
this center point will evenly divide the balance of the weight of the shoe
on either side of the lines. (Horseshoes with less weight in the heel calks
than other horseshoes either have less weight in the closed end of the
shoe or the center of gravity of the shoe is higher up towards the closed
end of the shoe). These lined divisions separating the balance of
the weight of the shoe on either side can be determined by finding the
balancing point of the horseshoe by setting the shoe up on the edge of
a three sided ruler or on the edge of a yardstick. Where the shoe
balances, lines can be chalked on the shoe and painted in some color scheme,
for easy reference while pitching.
9B ALL
OTHER LINES THROUGH THE CENTER POINT WILL EVENLY DIVIDE THE BALANCE OF
THE WEIGHT OF THE SHOE ON EITHER SIDE OF THE LINES
IMPORTANCE OF KNOWING WHERE THE CENTER OF GRAVITY IS
10 It is important to know where the center of the shoe is, because
it is the center of the shoe that all good horseshoe pitchers throw straight
at the stake at the opposite end that gives them such a high percentage
of ringers. They greet the stake, lining up the center of gravity
of the shoe with the stake when they aim (note: When aiming, the
center of gravity of the shoe will generally be to the right of a right
handed pitcher or to the left of a left handed pitcher, if the shoe is
being held at the same position at which the pitcher plans to release the
shoe at the end of the forward swing). The pitcher will swing straight
back with the center of the shoe during their back swing and they come
straight forward in their forward swing with the center of the shoe constantly
dead on an imaginary line between the center of the shoe and the stake
(remember we are discussing the good pitchers here!). At all times,
a good pitcher is consciously or sub-consciously aware of the path that
the center of the shoe is taking in the back swing and during the forward
swing, but most critically during the forward swing.
THROWING AN OPEN SHOE AT THE STAKE DOES NOT HAVE TO DEPEND ON LUCK
11 Whether or not the shoe is open (note it is only open on one
of the four sides of the average legal shoe) or closed when it arrives
at the stake can greatly depend on whether or not the pitcher gets lucky
or is unlucky. Or better yet, it will depend on whether or not the
pitcher put the proper turn rotation on the shoe during his forward swing
and properly pivoted his rotation turn around the center of gravity of
the shoe. (note: a shoe may not exceed 7-1/4 inches in width and
may not exceed 7-5/8 inches in length (the maximum length is the reason
the heel calks have to be heavier to make up for the open end of the shoe,
rather than make the shoe longer). The thickness may not exceed 1
inch and the open side may not be open more than 3-1/2 inches, with 3-5/8
inches allowed on used shoes with worn points from hitting too many ringers).
BACK SWINGS MAY VARY
12 It is not necessary that a pitcher’s back swing stays on the
imaginary line between the center of the shoe and stake where he greeted
the stake, as long as his forward swing is dead on this imaginary line.
Some pitchers are able to get back on the line in the last few inches of
their forward swing, but it is hard enough to stay on the line during the
whole forward swing, without adding the extra challenge of finding that
line in the last few inches of the forward swing before releasing the shoe.
I suspect that many of the great pitchers even prefer staying on that line
from the time they line up their shoe during the greeting, including staying
on the line during their back swing and through the entire forward swing
until the shoe is released.
STRAIGHT BACK SWING AND FORWARD SWING
13 Some good pitchers have been known to keep their shoe pretty
much in the same position during their whole forward swing (in other words
very close to the position it will be in at the release point) without
any turn rotation motion being applied and then in the last inch or so
before release “snap” their wrist to put a turn influence on the shoe.
The challenge of this method is that there is little margin for error and
the pitcher may not “snap” the wrist enough or “snap” it too much.
(This method also has a tendency to wear out a pitcher’s wrist, causing
them to give up horseshoe pitching and take up chess). It is much
more natural to start a smooth gradual turn of the shoe around the center
of gravity at the beginning of the forward swing or at least at the bottom
of the swing by the leg (or just after the shoe has cleared the leg).
Some would argue that starting it at the bottom by the leg is still not
enough time and distance for a turn rotation around the center of the shoe
to be consistent pitch after pitch and would advise beginning it at the
beginning of the forward swing. It also depends on whether a pitcher
is more comfortable beginning the gradual turn rotation at the beginning
of the forward swing, rather than trying to execute it at the point that
the shoe clears the leg. This is why some 1-1/4 clockwise pitchers
have the shoe cocked points slightly to the right if they are right handed,
or to the left if they are left handed, at the peak of their back swing,
so the shoe is pretty vertical when it passes the leg and then a 1/8 or
¼ turn rotation motion is applied around the center of gravity of
the shoe from the leg up to the point of release of the shoe.
HOW MUCH TURN ROTATION TO PUT ON A SHOE
14A How much rotation turn to start on the shoe while it is still
in the hand (which happens to be the only time a pitcher can really affect
the flight of the shoe, no matter how much he waves his hands after the
release of the shoe) is the big question and it depends on a few other
factors in the pitcher’s delivery. The turn the pitcher is throwing
in flight is one very important factor (3/4, 1-1/4, 1-3/4 or flip?).
The higher the pitcher throws the shoe the less turn motion needed because
it will travel just a little farther (two sides of the hypotenuse while
knowing the height of the release from the ground, plus the peak high point
of the shoe, using calculus could determine the actual distance traveled).
Even the length of the step is a factor (with a longer step usually used
by a low throwing pitcher and a shorter step usually used for a higher
throwing pitcher), plus the length of the step also determines the length
of the swing being used to influence the turn of the shoe around the center
of gravity of the shoe. Remember any turn influence applied to the
shoe is turning at so many degrees of the shoe (sixteenths, eighths or
quarters per so many feet. Illustrative example: A 1-1/4 shoe
thrown 36 feet from a starting point (maybe 1 foot past the foul line plus
3 feet step) of three feet or so off the ground may still travel 40 feet
with a given arch. There are 5 quarter turns in a pure 1-1/4 turn
thrown shoe. A pure 1-1/4 turn clockwise thrown shoe by a pitcher
will have the points of the shoe pointing away from the center of the shoe
(to the left for a right handed pitcher and to the right for a left handed
pitcher). For the shoe to turn exactly 5 quarters over a distance
of 40 feet requires that the pitcher put a turn rotation motion of ¼
turn per 8 feet (40 divided by 5 equals 8).
EFFECT OF THE STEP ON THE FORWARD MOTION OF THE SHOE
14B Another thing to know about here or to keep in consideration
is the effect of a short step versus the effect of a long step on the forward
motion of the horseshoe during the forward swing. First, let us consider
a swing and release of a shoe without any step at all. Since the
arm is attached to most pitcher’s shoulders, from the side of the leg,
a shoe takes a ¼ circular motion from the leg to a point where the
arm is straight out in front of the pitcher. The arm is a certain
length and the shoe will always remain a certain distance from the socket
in the shoulder of the pitcher, if the pitcher keeps the arm fully extended
during the entire forward swing from the leg to being parallel to the ground.
The arm does not have stretching ability to make the path of the shoe go
in a straight line from the bottom of the leg to the point of release.
But, when the pitcher begins to take a step (short or long) this lowers
the hand (and the shoe if it is still in the pitcher’s hand), so that it
takes a more straight path to the point of release. To help visualize
this principle: A pitcher who would literally do the splits while
taking the longest step possible, could actually make the shoe go straight
along the ground parallel with the ground at the same height that the shoe
was at the side of the leg. Some low throwing line drive pitchers
nearly do the splits and rely on pure strength to “fire” the shoe down
the court at this 1-1/2 foot to 2 feet level of height. They would
be taking what would be considered a long step to nearly doing the splits.
To map out the exact path a horseshoe would take while in the pitcher’s
hand from the leg to the point of release, the pitcher could have another
individual stand in the pit beside the pitcher and hold a measuring tape
at zero beside the center of the shoe when hanging down the side of the
pitcher. The pitcher could then take the step that feels most comfortable
and hold the shoe out in the extended position that it would be at the
point of release. The helper in the pit could then stretch the tape
and hold the extended end beside the center of the shoe at the release
point. This will make a straight line of tape, because the shortest
distance between two points is a straight line. While the helper
in the pit is standing there holding the tape, the pitcher could start
again in slow motion, taking the step so that the center of the shoe is
always right beside the rising tape until the arm is fully extended.
This will only impress on the pitcher that there is a timed step with a
certain swing of the shoe that will make the path of the shoe go straight
out to the point of release. It may not matter one way or the other
in the long run, but it is a good principle to understand and keep in mind
and if timed properly, the shoe will always be released at the exact end
of the step and if the step is the same pitch after pitch, then guess what?
The shoe will be released at the same point and height, pitch after pitch!
Wouldn’t that be a nice consistency to have?
HOW MUCH TURN FOR HOW LONG
15A Since the pitcher is not going to be swinging the shoe a
total of 8 feet during the forward swing and applying a gradual quarter
turn rotation motion on the shoe for 8 feet before release, then lets say
for the sake of illustration (we are getting the general picture here,
not splitting hairs or splitting inches) let’s say the pitcher normally
stands at the stake and takes about a 3 feet step before delivery.
If the shoe turn rotation around the center of gravity of the shoe is started
about the point of the pitcher’s leg, then the shoe will travel approximately
4 feet, with his 3 feet step and say an additional foot reach beyond the
extended foot (again rounding off to make the illustration of what is taking
place with the turn of the shoe). While influencing the turn of the
shoe while in the pitcher’s hand a distance of 4 feet, then the pitcher
would want to put about 1/8 turn rotation motion around the center of gravity
of the shoe from the lowest point beside the leg to the very point of release.
(Note: For the pitcher who wants the shoe to be level at the release
point,
this is why the expression “slightly cock the shoe” is used. A shoe
in vertical position beside the leg and level upon release would be taking
a full quarter turn in this approximate 4 feet of influence. A shoe
in vertical beside the leg, would only need about a 1/8 turn around the
center during the upward swing to the release point. Therefore the
vertical shoe at the side of the leg would need to be released in a slightly
cocked position approximately 45 degrees. Just remember, for a 1/8
turn for 4 feet, either slightly cocked from the leg to level upon release
or vertical from the leg to slightly cocked upon released.
WHAT AMOUNT OF INFLUENCE STAYS WITH THE SHOE INTO FLIGHT?
15B This turn motion must be gradual, because it is actually
the force on the shoe at the instant it is released that is carried into
flight with the shoe. If the hand turn rotation motion put on the
shoe is jerky, then only the influence in the last smooth continuous split
second before it is released will be on the shoe at release. When
a pitcher is nervous, say when trying to pitch two ringers for a won game
or just nervous for some other reason, everything a pitcher does tends
to be jerky. A smooth gradual turn of the shoe for all the forward
swing or part of the forward swing (say from the leg up) is only helping
to guide and establish the exact
rate of turn on the shoe at the very moment of release, which is the only
moment of influence which really influences the shoe! To prove
this point, try a nice smooth rotation turn motion on the shoe around the
center of gravity of the shoe for the whole forward swing and then about
the last foot, hesitate just a split second (jerky motion) and you have
interrupted all the preparation you had on the shoe for that last point
of release. Now only the motion you put on after the jerky interruption
will be on the shoe and the rate of turn for that last foot will be what
is on the shoe. The pitcher will almost have to get lucky to pick
up that same rate of turn after a jerky split second hesitation.
The shoe cannot remember or will not retain all that rotation the pitcher
was trying to put on before the pitcher made the jerky hesitation.
Now if after the slight jerky hesitation, the pitcher's arm swing did not
lose the rate of turn on the shoe, then the effect will not hurt the shoe,
because as stated above, "the
exact rate of turn on the shoe at the very moment of release, is the only
moment of influence which really influences the shoe!"
. . . AND THE IMPORTANCE OF THE FOLLOW THROUGH
15C This is why the follow through is so important. Guess
where the center of gravity of the shoe is going if the pitcher's follow
through is reaching for a point 6 inches to the left or right of the stake?
That is correct, the shoe will land (or go by) the stake 6 inches to the
left or right. (This is assuming the pitcher's grip spot on the shoe
at the moment of release in directly in line with the center of the shoe
and the stake at the opposite end. If a right handed pitcher's grip-release
point on the shoe is 1 inch to the right of the center of gravity of the
shoe at the point of release, then the follow through should also go up
the stake 1 inch to the right.) It is not because the pitcher's hand
influenced the shoe after it was released, but as stated above, the directional
influence on the shoe at the moment of release is all that matters, but
the follow through will be a continuation of where the shoe was coming
from before release. Speaking of bad habits, a bad habit would be
for a pitcher to try to change the direction of the pitching hand and arm
swing exactly at the point of release, because sometimes the pitcher would
start this change of direction a split second before the release.
And why would a pitcher want to disrupt a smooth continuous motion of a
swing anyway, just because the shoe has been released? Thus the smooth
follow through of the smooth forward swing. They go together like
cookies and milk.
FACTOR OF WHERE SHOE IS GRIPPED
16 Another factor that is not understood by many pitchers is
where the shoe is gripped in relation to the center of gravity of the horseshoe,
while the center is lined up with the stake upon release. If a right
handed pitcher throwing a “true” 1-1/4 turn (clockwise turn with the open
end pointing to the left at release) grips the shoe high or more towards
the closed end of the shoe with the center of gravity to the left and the
closed end to the right in relation to the pitcher’s grip, then the shoe
will pretty much turn itself if the pitcher puts no turn rotation on the
shoe during the forward swing, but merely lets the shoe “pull” out of the
hand on the release. The weight of the shoe to the left of center
will start the rotation and literally pull out of the pitcher’s hand, if
the pitcher is not trying to keep the shoe. The closer the right
handed pitcher grips the shoe down the blade so that the center of gravity
is in line with the stake and his grip, then the shoe will not turn itself
and his turn rotation motion will be the only influence on the shoe.
If the right handed pitcher grips the shoe so that the center of gravity
is to the right of his grip upon release of the shoe (gripping the shoe
down on the blade point), then it takes turn rotation motion to counter
the natural draw of the shoe to turn counter-clockwise. This all
comes into play if the release is caused by the shoe pulling out of the
hand with some fingers and thumb holding on slightly while the shoe pulls
out. The “let the shoe work or turn itself” pitcher need only decide
where they want the heel calks or points during the flight of the shoe
and then make sure the shoe is in this position at the point of release.
For example: if the pitcher wants the shoe pointing in the air with
the open end during the full turning of the shoe in flight, then that will
be the position of the shoe at the point of release. Technical principle:
If the 1-1/4 clockwise pitcher uses the “let the shoe work or turn itself”
feature of pitching because of holding the shoe “high” on the blade toward
the closed end of the shoe, but does not totally let the shoe pull out
of the hand, some minor amount of turn influence may have to be applied
to the center of gravity of the horseshoe or some will have to be applied
if the grip hold is close to the center of gravity of the shoe, a little
lower down and not fully using the “shoe turning itself” feature.
SHOE CAN WORK ITSELF OR TURN ITSELF
17 Good horseshoe pitchers who allow their shoe to “work itself”
or “turn itself” will not understand why any pitcher should have to work
on influencing their shoe with turn rotation motion during the forward
swing delivery. My answer to that is that not everyone has the ability
to hold a horseshoe high on the blade and complete the full backward and
forward swing with such a grip hold. I’ve tried it and I just haven’t
been able to do it myself. I will continue to experiment on this
feature and maybe some day learn to do it, but until that day arrives,
I and many others will have to understand how to influence the turn on
our shoes while holding the shoe lower down the blades.
18A
WHAT IS A WOBBLE IN A SHOE (PART 1)
18B
WHAT IS A WOBBLE IN A SHOE (PART 2)
18C SHOE
TILT AND SHOE LIFT (THE UPWARD PATH)
18D SHOE
TILT AND SHOE LIFT (THE DOWNWARD PATH)
18E SHOE
TILT AND SHOE LIFT (MY PERSONAL DELIVERY)
OTHER PRINCIPLES TO BE DISCUSSED
33 When I get the time to gather my thoughts more, I am going
to
add into this writing the pitching style of carrying the shoe with a bent
elbow versus the straight arm pendulum swing which I used throughout this
writing to keep everything on one plane of thinking. I plan to include
my thoughts on the center of gravity of the shoe visualized as a small
sphere round dot, rather than just a flat "penny" dot in the same plane
as the rest of the shoe. In other words, not all revolving force
on the metal of the shoe spins in the plane that includes the weight center
of all the metal around the shoe itself. Most pitchers are not able
to put just a circular motion of spin on a shoe in one plane, but actually
are passing metal of the shoe through many planes in relation to the center.
For in fact, this center of the shoe never revolves, in mathematical terms
it is a "point", which means it as no dimensions (a line is actually one
dimensional, a plane is two dimensional, while space is three dimensional
(time is often called the fourth dimension or where the space our revolving
object--or horseshoe--occupies in the rest of space at certain points in
time)), and it is the metal of the shoe that revolves around this center
of gravity point, whether in the same plane as the metal of the shoe before
any motion is applied or in other planes going through the center after
the shoe goes into motion. I have not written about the speed of
the forward swing and how it will effect all the other elements of pitching.
(I may not have the understanding at this time to explain the speed or
what effect it will have). I believe the rhythm that my first commenting
reader refers to allows the pitcher to achieve the right speed needed with
all the other influence the pitcher is putting on the delivery. With
as much as I tried to discuss above, I realize I've left many things out.
I'm merely thinking out loud and printing my thoughts as they come to mind
on this web page. I'm no authority on horseshoe pitching and I don't
claim to be. I certainly do not mean to sound like a "know it all"
simply because I'm up front and open with my thoughts as they come to mind.
I strive only to express them in an animated way, so others can follow
a line (or plane) of thinking on these matters and possibly point out flaws
or omissions. As I stated in the introduction, I hope to generate
counter-thought from others who have a much greater "instinctive" understanding
of the game than I, but may not have ever tried to do what I've attempted
here in expressing thoughts in print on the physics and mathematics of
pitching horseshoes. After my CONCLUSION below, I'm beginning to
include readers' responses and comments. Also, I would like to begin
links on this page to other pages where the physics, mathematics and techniques
of horseshoe pitching are discussed by others.
CONCLUSION
This ends my initial writing
on:
The Theory of the Physics and
Mathematics of Horseshoe Pitching
The physics of the turning shoe
and the mathematics of the alignment
How to pitch horseshoes with
nearly a 100% ringer average . . . in your mind
However flawed my thinking is on these things, I have at least made the effort to put these thoughts down onto paper. The foundation has been laid. The Internet now has a page that tries to make sense of it all using physics and mathematics. I will continuously be changing this writing as time goes on and I learn more about many of these theories on horseshoe pitching. Getting out in the spring and trying out some of these theories will certainly help. I hope the reader has enjoyed this presentation and has either absorbed some of the ideas in a helpful way or has been inspired to put together their own theories which may compliment this presentation or correct parts of it and hopefully share some of them with me. If I’ve exposed some secrets that some pitchers had long ago figured out for themselves, but had been reluctant to share with the rest of us, for fear that we would get better (at their expense), then I’m sorry. It is just my nature to try and figure out things that I am attracted to and share with others the things that I learn.
What we have showed here in the
final analysis is that to throw ringers, the pitcher need only, “throw
the shoe far enough, …throw the shoe open, …and throw the shoe at the stake.”
Hi Kenny: I cannot thank you enough for your time replying to my request.
I do not do this out of any dis-repect. My only concern is for us to be
putting out information that our fellow horseshoe pitchers can count on
as true and relevant.
Let me start by saying that your math as far as I can figure is dead on.
Where I have a problem is with the application as it pertains to the "
Alignment and where to stand to take advantage" I understand what your
saying in paragraph 28 as to where your shoe is in relationship to the
stake and all that. But in paragraph 29 you get to the point where you
have drawn a right angle to the line between the stakes. This is where
the problem starts for me. Again let me say that the math you've done here
is dead on.If I stand on the left side of the pitching platform on a 90
degree angle to the stake line with everything sguare, the distance increases
by about 3/8 of an inch. If I stand on the right side of the platform being
a right handed pitcher the distance increases by about 1 3/8 inches
The problem for me is that "we" as horseshoe pitchers do not stand on a
line 90 degrees to the stake line. If you would please un-hook our end
the line between the stakes and insert a pencil. Then mark a 40' RADIUS
line across both sides of the pitching platform. This is the line that
we stand on to address the stake at the other end. Now no matter where
you stand on the radius line the distance remains constant. The line between
the stakes is no longer relevant as each pitcher creates his or her own
line between their horseshoe and the stake at the other end. To prove this
we will build that horseshoe pitching machine that you spoke of,set it
up on the radius line,adjust it to pitch ringers and from then on no matter
where we push or pull the machine on the radius line it will continue to
pitch ringers with no further adjustment. There is no need to get back
to the stake center line. that line moves with us.
If your still not convinced then please think of it this way. Forget the
horseshoe stakes. Replace the stakes with basketball hoops, Install the
3 point line ( RADIUS) and shoot baskets. No matter where you are on the
line the shot is always the same. the center line means nothing.
Again let me thank you for your time and let me further say that your work
on the center of gravity of a horseshoe is nothing short of spectacular.
I would hope that your work on this would inspire all horseshoe makers
to show us where the center of gravity is on their shoes when listing them
for
sale. Just think of the difference between a Imperial Maxx and a Diamond
super ringer or a Viper and a Ted Allen. Please keep up the great work
your doing and happy pitching.
Go to paragraph 29 to which Mr. Coles is
referring.
Your horseshoe friend
Bill Coles
Carthage, North Carolina
CHALLENGE ACCEPTED!
In August 2008, I received an address link for another web site blog
dedicated to finding the perfect horseshoe swing delivery. Bob Rasmussen
writes in detail about many of the things discussed on these pages, plus
he takes it a step further by explaining the equipment he has developed
to assist in training the muscles to duplicate the same motion over and
over. His blog web site is located at: http://tinyurl.com/MyWay1
. "Horseshoes My Way ... the
Search for My Perfect Swing" (in 7 parts). This
blog reads very well and fluent. Bob has done a lot of thinking and
planning for this presentation and I think it will be very beneficial for
all who visit.
LETTER FROM A HOOSIER PITCHER
Hello! I was reading the mathematics and physics
of pitching that you have on the web site, and found it informative, I
do have a degree from ISU, with a minor in physics. I think the way
you changed your delivery is on the right track. I will share what
I was taught about pitching and alignment.
I was taught that if you get the shoe up high enough after a fairly level release, it does not have to be perfectly level, the shoe will turn on its own to be open at the stake with either a turn and one fourth or a turn and three fourths. To get alignment I was taught to line up the knuckle of my thumb with the point on the stake I am pitching at when releasing the shoe. My knuckle is in the middle of the shoe, and if the shoe is high enough, it will be open and make a ringer like that every time.
I pitch Allens, but every shoe is balanced different with
ringer savers and so forth. My main problem is alignment because
my eyesight is not what it used to be. I am not an expert pitcher,
I thought I might share some things I was taught by my uncle and Clarence
Bellman. So I guess to sum up one could say that in the forward swing
if the shoe is lined up and at 7-8 feet in the air, it will rotate enough
to be open, maybe not perfectly open, but open enough to be a ringer.
You get the distance by the back swing and practice, practice. I
don't know if this will help anybody pitch any better, but this is the
way I pitch, and like I said, I am not an expert.
GP - IN 12/13/05
(follow-up letter)
I can explain exactly the way I deliver a shoe.
I bring it up to eye level flat, turn it inward, in line with the stake,
and start the back swing straight back from the stake. As it goes
past my leg in the back swing, I step forward like I am taking a long step,
but not a really long step. My back swing is about shoulder high, and after
the shoe goes past my leg in the forward swing, I roll my arm so the shoe
is fairly level when the knuckle of my thumb is about halfway up on the
stake. Then I release the shoe, which does not have to be perfectly
level, if it is fairly level, it will be fairly level at the stake, when
it goes on, if it does. If you chop off the back swing you will be
short, and if you swing back too far you will be out of line, or off balance
and step out of line. It is tricky to get it just right the way you
feel comfortable with it.
I think you will find with the thumb in the middle of
the shoe when you bring it up and the thumb in line with the stake, your
alignment will get better, and by practicing the back swing and forward
swing, you can improve up to 45-50% within a year. Keep balanced
in the forward step and be smooth with the delivery when you find out how
far back you have to swing to get the distance and be comfortable with
it. I found that Allens work best for this type of delivery, but
Walter Ray throws Clydesdales and uses pretty much the same delivery that
I do. If he is at Danville again this year, you might watch him pitch.
They don't call him Dead-eye for nothing. I saw him beat Alan at
Danville a few years ago, it was a heck of a game. Dead-eye is the
one who is the expert. Well, I'll close for now, have good Christmas
and HAPPY NEW YEAR.
GP - IN 12/14/05
Kenny Wolf's Open Letter Response to Walter Ray Williams,
Jr.
Of course I had you in mind when I wrote this page. With your cross knowledge of both horseshoe pitching and physics, I, like many others, wished that you had written extensively on the physics and mathematics of horseshoe pitching as you apparently did on the sport of bowling. (I also see that on your site, you offer a video you made on bowling for fellow bowlers). I also thought it would be very difficult to put horseshoe pitching into words, but I gave it a try here. I've stated many times on this page that I am not a great horseshoe pitcher and I have been very up front with that fact. You say that I use some physics terms in my description of how to pitch a horseshoe, but that I really don't use them properly. I do wish you had taken a sentence or two to give an example or two of my misuse of some physics terms. I want to know where I have erred, so I can get this page as close to the truth as possible. I have revised a couple of sections since I first put this page up. One section was on the "wobble" of a shoe. I realized I was wrong originally and I attempted to highlight my error in thinking and correct my statements on it. After receiving a letter from an individual asking me about my sections on the "tilt path" of a pitched horseshoe, I thought about his question and revised my thinking on this and highlighted my writing on this subject with a preface stating that it was not practical with the way most pitchers fly a horseshoe.
My main focus in using physics on this page was to instill an awareness in other horseshoe pitchers that a horseshoe has a center of gravity. This is a concept that I read from a fellow horseshoe pitcher on the internet. I didn't think of it myself! He wrote me after reading this page and his letter is printed below and signed as D. G. (Duane Goodrich). I don't think Mr. Goodrich would mind me crediting him with bringing this matter to my attention. I'm sure he would say that he was taught this concept by someone else, who learned it from someone else, and on and on into the past. I don't think discussing a horseshoe's "center of gravity" is using physics terms improperly. Keeping the center of gravity of a horseshoe on a straight line to the stake does not seem to me to be using physics terms improperly. I also emphasize different ways of putting a turn on the horseshoe. If the shoe is flying a distance of approximately 40 feet, give or take a few feet, I don't see how describing the amount of turn influence on the shoe for the approximate length of 8 feet to 4 feet (or the last 2 feet to 1 foot that it is in the hand) is using physics terms improperly? These simple physics principles are the main concepts delved into on this page!
In your response to Jim, I think you hit the nail on the head when you
said that "like most sports,
it comes down to repetition and feel." I agreed with you 100% when
I read that comment. But some of us struggle to come up with that
"feel" that great pitchers like you have discovered and some of us struggle
with "repetition" which come so natural to pitchers like you, Curt Day,
Elmer Hohl, Dan Kuchcinski, Mark Seibold, Alan Francis, Brian Simmons,
Ted Allen, Fernando Isais and the great Guy Zimmerman (only to mention
a few of our pitching idols and heroes). Some of us, like Jim from
Jackson's Gap, AL are looking for systematic structured advice from some
of our living great horseshoe pitchers on the physics and mathematics of
horseshoe pitching, so we know in our minds what the physics are that we
are trying to apply and so our practicing will not be in vain so often
as it is for some of us. We may never be able to fully apply this
valued knowledge, but we would like to see it presented by one or more
of the great living horseshoe pitchers. That was the purpose of my
page here! I'm not a great horseshoe pitcher and I don't have a degree
in physics, but I don't find it "very difficult to put some things into
words" once I take the time to work out the thoughts in my mind.
Walter Ray, because I greatly admire your skills and the time you take
with horseshoe pitching enthusiasts, I would like nothing more than to
see you write a treatise on the physics and mathematics of horseshoe pitching.
Maybe you would be surprised to find that you would express more things
the way I have here; and then again, maybe you would use a whole different
approach and vocabulary of physics terms. But either way, it would
be one of your greatest contributions to the sport. And you have
already contributed so much.
Thank you for commenting on this web site. Now get your 1-1/4
turn figured out again by physics or "repetition and feel" and go out there
and win some more horseshoe pitching world championships.
Sincerely and gratefully,
Kenny Wolf
Kenny Wolf
Curt
Day interview on how to pitch horseshoes
interviewed by his
son Paul Day
The Horseshoe Pitching
Career
and Tournament Results of Curt
Day
Frankfort, Indiana
Return to Curt Day Horseshoe Courts
Main Page